【刷题】DFS

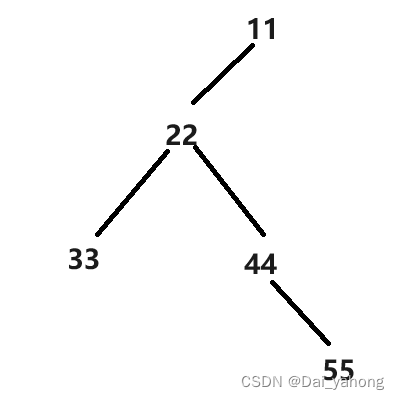
本文介绍: 给定一个二叉树的根节点 root，想象自己站在它的右侧，按照从顶部到底部的顺序，返回从右侧所能看到的节点值。输入: [1,2,3,null,5,null,4]输入: [1,null,3]输出: [1,3,4]

DFS

递归：
1.判断是否失败终止
2.判断是否成功终止，如果成功的，记录一个成果
3.遍历各种选择，在这部分可以进行剪枝
4.在每种情况下进行DFS，并进行回退。

199. 二叉树的右视图

给定一个二叉树的根节点 root，想象自己站在它的右侧，按照从顶部到底部的顺序，返回从右侧所能看到的节点值。
示例 1:
在这里插入图片描述
输入: [1,2,3,null,5,null,4]
输出: [1,3,4]
示例 2:
输入: [1,null,3]
输出: [1,3]
示例 3:
输入: []
输出: []

class Solution {
public:
    vector&lt;int&gt; rightSideView(TreeNode* root) {
        unordered_map<int, int&gt; rightmostValueAtDepth;
        int max_depth = -1;

        stack<TreeNode*> nodeStack;
        stack<int> depthStack;
        nodeStack.push(root);
        depthStack.push(0);

        while (!nodeStack.empty()) {
            TreeNode* node = nodeStack.top();nodeStack.pop();
            int depth = depthStack.top();depthStack.pop();

            if (node != NULL) {
            	// 维护二叉树的最大深度
                max_depth = max(max_depth, depth);

                // 如果不存在对应深度的节点我们才插入
                if (rightmostValueAtDepth.find(depth) == rightmostValueAtDepth.end()) {
                    rightmostValueAtDepth[depth] =  node -> val;
                }

                nodeStack.push(node -> left);
                nodeStack.push(node -> right);
                depthStack.push(depth + 1);
                depthStack.push(depth + 1);
            }
        }

        vector<int> rightView;
        for (int depth = 0; depth <= max_depth; ++depth) {
            rightView.push_back(rightmostValueAtDepth[depth]);
        }

        return rightView;
    }
};

给你一个无重复元素的整数数组 candidate s 和一个目标整数 target ，找出 candidate s 中可以使数字和为目标数 target 的所有不同组合，并以列表形式返回。你可以按任意顺序返回这些组合。
candidate s 中的同一个数字可以无限制重复被选取。如果至少一个数字的被选数量不同，则两种组合是不同的。
对于给定的输入，保证和为 target 的不同组合数少于 150 个。
示例 1：
输入：candidate s = [2,3,6,7], target = 7
输出：[[2,2,3],[7]]
解释：
2 和 3 可以形成一组候选，2 + 2 + 3 = 7 。注意 2 可以使用多次。
7 也是一个候选， 7 = 7 。
仅有这两种组合。
示例 2：
输入: candidate s = [2,3,5], target = 8
输出: [[2,2,2,2],[2,3,3],[3,5]]
示例 3：
输入: candidates = [2], target = 1
输出: []

class Solution {
public:
    void dfs(vector<int>&amp; candidates, int target, vector<vector<int>>&amp; ans, vector<int>&amp; combine, int index) {
        if (index >= candidates.size()) return;
        if (target==0) {
            ans.emplace_back(combine);
            return;
        }
        dfs(candidates, target, ans, combine, index+1);
        if (candidates[index]<=target){
            combine.push_back(candidates[index]);
            dfs(candidates, target-candidates[index], ans, combine, index);
            combine.pop_back();
        }
    }

    vector<vector<int>> combinationSum(vector<int>&amp; candidates, int target) {
        vector<vector<int>> ans;
        vector<int> combine;
        dfs(candidates, target, ans, combine, 0);
        return ans;
    }
};