每日一练：LeeCode-102、二又树的层序遍历【二叉树】

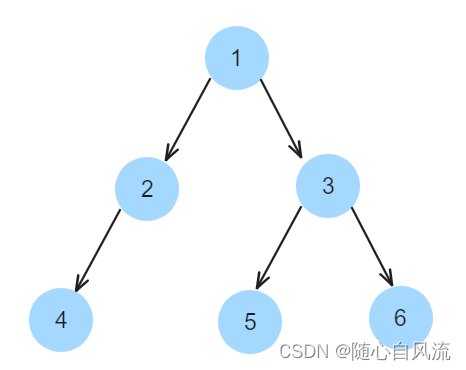
本文介绍: 给你二叉树的根节点 root ，返回其节点值的层序遍历。输入：root = [3,9,20,null,null,15,7]输出：[[3],[9,20],[15,7]]输入：root = [1]输出：[[1]]输入：root = []输出：[]树中节点数目在范围[0, 2000]内。

本文是力扣LeeCode-102、二又树的层序遍历学习与理解过程，本文仅做学习之用，对本题感兴趣的小伙伴可以出门左拐LeeCode。

给你二叉树的根节点 root ，返回其节点值的 层序遍历 。（即逐层地，从左到右访问所有节点）。

示例 1：
在这里插入图片描述

输入：root = [3,9,20,null,null,15,7]
输出：[[3],[9,20],[15,7]]

示例 2：

输入：root = [1]
输出：[[1]]

示例 3：

输入：root = []
输出：[]

提示：

树中节点数目在范围 [0, 2000] 内
-1000 <= Node.val <= 1000

思路

层序遍历，符合队列queue 先进先出 的规律：左边先进，左边先出，只要保证从上到下每层遍历即可

代码实现

下列两段代码可以作为BFS模版 解决二叉树层序遍历相关的问题
BFS模版1

class Solution {
    public List<List<Integer>> levelOrder(TreeNode root) {
        List<List<Integer>> res = new ArrayList<>();
        Queue<TreeNode> queue = new LinkedList<>();
        
        if(root==null) return res;
        queue.add(root);
        while(!queue.isEmpty()){
            int size = queue.size();
            List<Integer> tempList = new ArrayList<>();
            // 这⾥⼀定要使⽤固定⼤⼩size，不要使⽤que.size()，因为que.size是不断变化的
            for(int i=0;i<size;i++){
                TreeNode node = queue.poll();
                tempList.add(node.val);
                if(node.left!=null)queue.add(node.left);
                if(node.right!=null)queue.add(node.right);
            }
            res.add(tempList);	//每层遍历完，放进结果即可
        }
        return res;
    }
}

BFS模版2

class Solution {
    public List<List<Integer>> levelOrder(TreeNode root) {
        List<List<Integer>> res = new ArrayList<>();
        if(root==null){return res;}

        int start = 0; 
        int end = 1; 
        Queue<TreeNode> queue = new LinkedList<>();
        queue.add(root);
        List<Integer> tempList = new ArrayList<>();
        while(!queue.isEmpty()){
            TreeNode t = queue.poll(); 
            tempList.add(t.val);
            start++;

            if(t.left!=null) queue.add(t.left);
            if(t.right!=null) queue.add(t.right);
            if(start == end){	//某层都遍历完后，才添加结果，并初始化
                res.add(new ArrayList<>(tempList));
                start = 0;
                end = queue.size();
                tempList.clear();
            }
        }
        return res;
    }
}