leetcode – 1329. Sort the Matrix Diagonally

本文介绍: leetcode – 1329

Description

A matrix diagonal is a diagonal line of cells starting from some cell in either the topmost row or leftmost column and going in the bottom-right direction until reaching the matrix’s end. For example, the matrix diagonal starting from mat[2][0], where mat is a 6 x 3 matrix, includes cells mat[2][0], mat[3][1], and mat[4][2].

Given an m x n matrix mat of integers, sort each matrix diagonal in ascending order and return the resulting matrix.

Example 1:
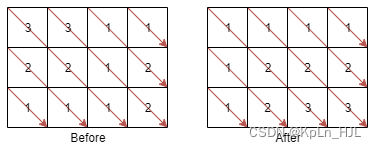
在这里插入图片描述

Input: mat = [[3,3,1,1],[2,2,1,2],[1,1,1,2]]
Output: [[1,1,1,1],[1,2,2,2],[1,2,3,3]]

Example 2:

Input: mat = [[11,25,66,1,69,7],[23,55,17,45,15,52],[75,31,36,44,58,8],[22,27,33,25,68,4],[84,28,14,11,5,50]]
Output: [[5,17,4,1,52,7],[11,11,25,45,8,69],[14,23,25,44,58,15],[22,27,31,36,50,66],[84,28,75,33,55,68]]

Constraints:

m == mat.length
n == mat[i].length
1 <= m, n <= 100
1 <= mat[i][j] <= 100

Solution

For the diagonal, if the nodes are in the same diagonal, they have the same x-y. Iterate all the numbers in the same diagonal, and sort, and fill them back.

Time complexity:

(

∗

log

⁡

(

∗

)

o(m*n*log (m*n))

$o (m * n * lo g (m * n))$
Space complexity:

(

∗

)

o(m*n)

$o (m * n)$

Code

class Solution:
    def diagonalSort(self, mat: List[List[int]]) -> List[List[int]]:
        diag_numbers = {}
        m, n = len(mat), len(mat[0])
        # get diag numbers
        for x in range(m):
            for y in range(n):
                diag_key = x - y
                if diag_key not in diag_numbers:
                    diag_numbers[diag_key] = []
                diag_numbers[diag_key].append(mat[x][y])
        # sort
        for k, v in diag_numbers.items():
            diag_numbers[k] = sorted(v, reverse=True)
        # fill back
        for x in range(m):
            for y in range(n):
                mat[x][y] = diag_numbers[x - y].pop()
        return mat